probability distribution function particle in a box The probability of finding a particle a certain spot in the box is determined by squaring \(\psi\). The probability distribution for a particle in a box at the \(n=1\) and \(n=2\) energy levels looks like this: $16.99
0 · probability distribution of quantum particle
1 · probability distribution of particle
2 · probability density of particle
3 · probability density in a box
4 · probability density distribution
5 · probability density 1d box
6 · particle in a box diagram
7 · 1 dimensional box particle probability
5 axis 6'x10' water jet cutter. This waterjet cutting machine comes with CNC controller and software, 30HP pump and abrasive hopper. Very low hours and in Excellent condition. Power .
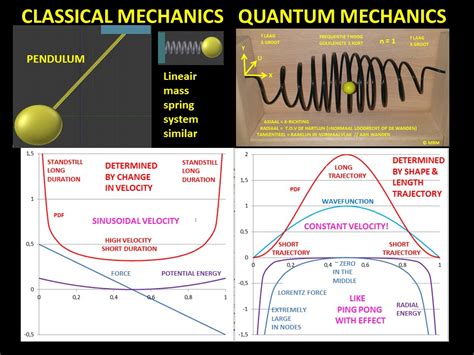
This principle states that for large quantum numbers, the laws of quantum physics must give identical results as the laws of classical physics. To illustrate how this principle works for a quantum particle in a box, we plot the probability density .
The probability of finding a particle a certain spot in the box is determined by squaring \(\psi\). The probability distribution for a particle in a box at the \(n=1\) and \(n=2\) energy levels looks like this:
Figure \(\PageIndex{3}\): The probability density distribution \(|\psi_n(x)|^2\) for a quantum particle in a box for: (a) the ground state, \(n = 1\); (b) the first excited state, \(n = 2\); and, (c) the nineteenth excited state, \(n = .
To illustrate how this principle works for a quantum particle in a box, we plot the probability density distribution \[|\psi_n(x)|^2 = \dfrac{2}{L} sin^2 (n\pi x/L) \label{7.50} \] for finding the particle around location \(x\) between the walls .
The probability distribution for a particle in a box at the \(n=1\) and \(n=2\) energy levels looks like this: Notice that the number of nodes (places where the particle has zero probability of being located) increases with increasing energy n.
In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes the movement of a free particle in a small space surrounded by impenetrable barriers.The first five wave functions for a particle in a box are shown. The probability of finding the particle near x = L/2 is. least for n = 1. least for n = 2 and n = 4. least for n = 5. the same (and nonzero) .Particle in a Box Outline - Review: Schrödinger Equation - Particle in a 1-D Box. Eigenenergies. Eigenstates. Probability densities The probability (P) can be calculated by integrating the probability distribution (P (x)) over an interval . Probability of Finding a Value within an Interval. {\displaystyle P=\int \limits _ .
In this Insight, I’ll briefly outline why the particle in a box problem is important, what the solutions mean, and what the solution to higher dimensional (2-D) boxes looks like. Along the way, you might even pick up some useful .In quantum mechanics, the properties of a particle are described by its wave function, a mathematical function that encodes information about the likelihood of particle properties like its location and momentum. . In a one-dimensional box, the probability distribution is constrained by the physical conditions of the box and the boundary . The momentum‐space probability distribution functions, |\(\Phi\)(n,p)| 2, for the n = 1, 4, 6, 8 and 10 energy levels of the particle in a one‐dimensional box are displayed below. They show the probability that the .
The 1D Particle in a Box Problem. The 1D particle in a box simplifies the quantum mechanical model that describes the behavior of a single particle confined in a box, just as the electrons are .Consider the particle in a 1-d box, we know very well the solutions of it. I'd like to see how the correspondence principle will work out in this case, if we consider position probability density function (pdf) of the particle.That is to say, this wave function, a linear sum of wave functions corresponding to different energies, has a probability distribution that sloshes back and forth in the box: and, any attempt to describe a classical-type particle motion, bouncing back and forth, necessarily involves adding quantum wave functions of different energies.Figure \(\PageIndex{1}\): The potential energy function that confines the particle in a one-dimensional box. Solutions \(\psi(x)\) to this equation have a probabilistic interpretation. In particular, the square \(|\psi(x)|^2\) represents the probability density of finding the particle at a particular location x. This function must be integrated .
The index n is called the energy quantum number or principal quantum number.The state for is the first excited state, the state for is the second excited state, and so on. The first three quantum states (for of a particle in a box are shown in .. The wave functions in are sometimes referred to as the “states of definite energy.” Particles in these states are said to occupy energy levels .
williams metal fabrication
Within the box. the wave function Y. is an eiaenfunction - of the kinetic-energy operator, with eigenvalue Based on these observations, it is tempting to conclude . probability distribution for the particle in state Y, in the momentum space. Just as the probability density to find the particle located within the infinitesimal range dz about
The probability of finding a particle in a 1D-Box (from the classical view, the particle behaves as a particle but not a wave) is the same everywhere in the box. . the probability distribution will be a delta function. But if there's any uncertainty in any of these quantities, then the probability distribution will be wider.A particle in a box is in a state of definite energy. The probability distribution function for this state Group of answer choices depends on the particular state of definite energy varies with time, but the variation is not a simple oscillation oscillates in time, with a frequency that depends on the size of the box does not vary with time.
probability distribution of quantum particle
Consider a particle in a box with infinite barriers. By solving the Schrödinger we can find the probability of finding the particle at some points in the box. How can we find the probability of par.A particle in the three-dimensional box shown in the given figure is in the state n X = 2, n Y = 1, n Z = 3 n_X=2, n_Y=1, n_Z=3 n X = 2, n Y = 1, n Z = 3. Find the planes (other than the walls of the box) on which the probability distribution function is zero. Tour Start here for a quick overview of the site Help Center Detailed answers to any questions you might have Meta Discuss the workings and policies of this site
For the following state of a particle in a three-dimensional box, at how many points is the probability distribution function a maximum: nX= 2, nY= 2, nZ = 1? There are 3 steps to solve this one. SolutionQuestion: A particle is in a three-dimensional cubical box that has side length L. For the state nx= 3, ny = 2 and nz = 1 for what planes (in addition to the walls of the box) is the probability distribution function zero?For the following states of a particle in a threedimensional box, at what points is the probability distribution function a maximum: n X = 2 n_X=2 n X = 2, n Y = 2, n Z = 1? n_Y=2, n_Z=1 ? n Y = 2, n Z = 1? In these notes on statistical thermodynamics (pp. 62), I encountered this [topic: particle in a 1D box]: We shall adopt the initial condition that the probability distribution function has the.
The wave describing the quantum particle is reflected at the boundaries, a phenomenon analogous to the reflection of light by a mirror. In general the uncertainty in the position of the particle changes with time. FOOTNOTE 1 The following animations show that as time goes on the probability distribution spreads over the whole box.\({ }^{*}\) A particle is in the ground state of a box of length \(L\). Suddenly the box ispands (symmetrically) to twice its size, leaving the wave function undisturbed. Show that the probability of finding the particle in the ground state of the new box is \((8 / 3 \pi)^{2}\).Draw the wave function for a particle in a box at the n=4 energy level. 2. Draw the probability distribution for a particle in a box at the n=3 energy level. 3. What is the probability of locating a particle of mass m between x=L/4 and x=L/2 in a 1-D box of length L? Assume the particle is in the n=1 energy state. 4. Suggest where along the box
Answer to For the following state of a particle in a. Science; Advanced Physics; Advanced Physics questions and answers; For the following state of a particle in a three-dimensional box, at how many points is the probability distribution function a maximum: nX= 2, nY= 2, nZ = 1?Here ψ1(x) and ψ3(x) are the normalized stationary-state wave functions for the n = 1 andn = 3 levels, and E1 and E3 are the energies of these levels. The wave function is zero for x<0 and for x>L. Part A. Find the value of the probability distribution function at x=L/2 as a function of time.Answer to * The classical probability distribution function. Science; Advanced Physics; Advanced Physics questions and answers * The classical probability distribution function for a particle in a onedimensional box on the x axis in the region of P(x)=1L(:x:)=12L(:x2:)=13L20 is given by P(x)=1L.
Statistics and Probability; Statistics and Probability questions and answers; For the following state of a particle in a three-dimensional box, at how many points is the probability distribution function a maximum: nx=2, ny=2, nz = 1? ΤΕΙ ΑΣφ N = 1 Submit Previous Answers Request Answer X Incorrect; Try Again; 4 attempts remainingDraw the wavefunction for a particle in a box at the n=5 energy level. 2. Draw the probability distribution for a particle in a box at the n=4 energy level. 3. What is the probability of locating an electron between L/4 and L/2 in a box of length L? Assume the n=1 energy state. 4. Calculate the electronic transition energy of aspirin assuming a .First of all, I assume that you know that the probability (density) distribution is given by the squared amplitude of the wavefunction. In your example, you are given the wavefunction in the position basis, so it gives you the position probability density. If you want the momentum probability density, you have to change basis to get $\psi(p)$.. The standard way to change .
The particle-in-a-box model provides a reasonably accurate description of the energies of the π electrons of linear conjugated molecules such as linear conjugated alkenes. The general chemical structure of an unsubstituted linear conjugated alkene with 2 k carbon atoms and hence k double bonds, where k is an integer, is shown below. (a) Sketch the probability distribution as a .
probability distribution of particle
probability density of particle
Great for at the or at the beach. Drink your water AND store your valuables at the same time! Also the body is vacuum and keep water warm and cold at least 12 hours. Water Storage Space Size: 2.2*2.2*3.9 inches. Valuables Storage Space Size: 2.8*2.8*3.9 inches.
probability distribution function particle in a box|1 dimensional box particle probability